Multiplying and Dividing Positive and Negative Integers

Hello,

In our last post, we discussed how to add and subtract positive and negative integers.  Today, we are going to learn how to multiply and divide by positive and negative integers.  It is basically the same thing as multiplying and dividing normally, but we need to memorize some rules so we do not mix any signs up.

Rules to remember:

1.) Positive x positive = positive
2.) Negative x negative = positive
3.) Positive x negative = negative
4.) Negative x positive = negative
5.) Positive / positive = positive
6.) Negative / negative = positive
7.) Positive / negative = negative
8.) Negative / positive = negative

***Same sign = positive****
***Different sign = negative***

Get it? Let's practice!

Examples:

1.) (-5) x (-6) = ?
2.) (4) x (-2) = ?
3.) (9) x (9) = ?
4.) (-3) x (12) = ?
5.) (-36) / (9) = ?
6.) (80) / (-10)  = ?
7.) (-12) / (-6) = ?
8.) (56) / (7) = ?
9.) (12) x (-6) = ?
10.) (-11) / (11) = ?

Solutions:

1.) 30
2.) -8
3.) 81
4.) -36
5.) -4
6.) -8
7.) 2
8.) 8
9.) -2
10.) -1

The following links will help reiterate our lesson and will help you practice multiplying and dividing integers with negative signs:

1.) Examples and explanation with multiplying and dividing positive and negatives
2.) Practice worksheet with multiplying positive and negative integers; Answers at the bottom
3.) Practice worksheet with dividing positive and negative integers; Answers at the bottom

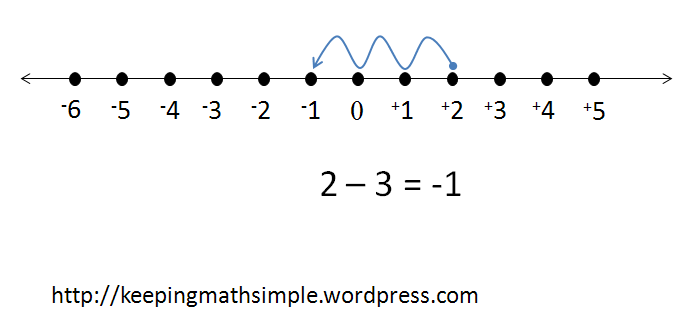
Adding and Subtracting Positive and Negative Integers

Hello,

We are going to move on from measurement, and we are going to learn how to add and subtract positive and negative integers.  Basically, you just need to remember some basic rules and you will be fine.  

The following are rules that you should memorize, especially when you get into more advanced math, which requires you to remember adding and subtracting positive and negative numbers very quickly:

1.) positive + positive = positive; add the two numbers like you normally would

2.) Larger positive + smaller negative = positive; subtract the two numbers like you normally would

3.) Smaller positive + larger negative = negative; subtract the two numbers like you normally would

4.) negative + negative = negative; keep the negative and add the two numbers like you normally would

5.) positive - positive = positive; subtract the two numbers like you normally would

6.) positive - negative = positive + positive = positive; just add the two numbers like you normally would

7.) negative - negative = negative or a positive depending; subtract the two numbers like you normally would 

This may look extremely confusing, but let's try some examples and hopefully it will look less confusing!

Examples:  

1.) 8 + 2 = ?

2.) 5 + (-10) = ?

3.) 4 - 9 = ?

4.) (-6) + (-6) = ?

5.) (-7) - (-3) = ?

6.) 10 - 5 = ?

7.) (-2) + 14 = ?

8.) 8 - 12 = ?

9.) 1 + (-4) = ?

10.) 3 - (7) = ?

Solutions:

1.) 8 + 2 = 10

2.) 5 + (-10) = -5 ; the larger number has the negative, so we are using rule 3 from above; we keep the negative and subtract like normal.

3.) 4 - 9 = -5 ; this can also be written as 4 + (-9) and it becomes just like number 2; we keep the negative and subtract the two.

4.) (-6) + (-6) = -12 ; when we add two negatives, we keep the negative and add the two numbers together.

5.) (-7) - (-3) = (-7) + 3 = -4 ; a minus a negative is equal to a positive, and since the negative is with our bigger number, 7, we keep the negative and subtract the two.

6.) 10 - 5 = 5

7.) (-2) + 14 = 14 - 2 = 12

8.) 8 - 12 = 8 + (-12) = - 4

9.) 1 + (-4) = -3

10.) 3 - (7) = -4 

The following are links that help reiterate our lesson, and they will also help you practice:

1.) Reiteration of our lesson, with a practice at the bottom

2.) Another reiteration of how to add and subtract positive and negative integers

3.) Example problems with adding and subtracting positive and negative integers using a number line

4.) Another practice worksheet

I hope these help!

Measurement in Terms of Volume: Metric System

Hello,

So far, we have learned units of the metric system in terms of length, including meters, millimeters, centimeters, kilometers, yards, feet, and inches.  Today, we are going to be learning units of the metric system in terms of volume.  Volume is defined as the amount of space that an object or substance takes up measured in cubic units.  The basic unit of volume is the liter, just as with length, we had the basic unit of length being the meter.

We will define 6 prefixes that contain liter, and then we will learn how to convert from each.

Prefixes of the Liter:


1.) Kiloliter ; kilo = 1000 liters
2.) Hectoliter ; hecto = 100 liters
3.) Decaliter ; deca = 10 liters
4.) Deciliter ; deci = 1/10 liters
5.) Centiliter ; centi = 1/100 liters
6.) Milliliter ; milli = 1/1000 liters

Notice the pattern above; as we move down from 1 through 6, the unit is one-tenth as large as the previous unit.  If we memorize these prefixes, it is very easy to convert from one unit to the other.  Let's practice converting each to liters and vice versa.

Examples:   


1.) 20 hectoliters = ? liters
2.) 14 kiloliters = ? liters 
3.) 4 liters = ? milliliters
4.) 32 liters = ? decaliters
5.) 2 Centiliters = ? liters
6.) 45 liters = ? milliliters 
7.) 8 deciliters = ? liters
8.) 50 kiloliters = ? liters
9.) 5 liters = ? decaliters
10.) 6 hectoliters = ? liters

Solutions:


1.) 20 hectoliters = 2000 liters ; 20 x 100 = 2000
2.) 14 kiloliters = 14000 liters ; 14 x 1000 = 14000
3.) 4 liters = 0.004 liters ; (1/1000) x 4 = 0.004
4.) 32 liters = 3.2 decaliters ; 32/10 = 3.2
5.) 2 centiliters = 0.02 liters ; (1/100) x 2 = 0.02
6.) 45 liters = 45000 milliliters ; 45/(1/1000) = 45000
7.) 8 deciliters = 0.8 liters ; (1/10) x 8 = 0.8
8.) 50 kiloliters = 50000 liters ; 50 x 1000 = 50000
9.) 5 liters = 0.5 deciliters ; 5/10 = 0.5
10.) 6 hectoliters = 600 liters ; 6 x 100 = 600

Get it? The following links will help you practice with these conversions:

1.) worksheet to help you practice converting from milliliters to liters and vice versa
2.) Answer key to worksheet 1
3.) Worksheet: a nice review of conversions involving length and volume
4.) Answer key to worksheet 2

This link below will help reiterate units of length and volume

4.) Charts for units of length and volume

This link below is meant to be fun: Metric word search =) Do not cheat and look at the answers at the bottom of the page, try it yourself first:

5.) Metric Word Search with Solutions at the Bottom of the Page

Measurement in Terms of Length: Metric System

Hello,

In our previous post we talked about measurements of the metric system in terms of length, including converting measurements to meters.  Today, we are going to learn about more units of the metric system in terms of length.  We are going to focus on inches, feet, and yards.  We are going to learn how to convert from the three.

In order to make conversions, we must note:

1.) 1 inch = 0.83333333... feet
2.) 1 inch = 0.0277777... yards
3.) 1 foot = 12 inches
4.) 1 foot = 0.333333... yards
4.) 1 yard = 36 inches
5.) 1 yard = 3 feet

Let's try some examples:

1.) 4 feet = ? inches
2.) 15 inches = ? feet
3.) 5 yards = ? feet
4.) 8 feet = ? yards
5.) 20 inches = ? yards
6.) 45 yards = ? inches
7.) 6 feet = ? inches
8.) 36 inches = ? yards
9.) 12 yards = ? feet
10.) 80 inches = ? feet

Solutions:

1.) 4 feet = 48 inches ; 4 x 12 = 48
2.) 15 inches = approximately 12.5 feet ; 15 x 0.8333333 = 12.49....
3.) 5 yards = 15 feet ; 5 x 3 = 15
4.) 8 feet = approximately 2.7 yards ; 8 x 0.33333 = 2.66....
5.) 20 inches = approximately 0.6 yards ; 20 x 0.027777 = 0.55....
6.) 45 yards = 1620 inches ; 45 x 36 = 1620
7.) 6 feet = 72 inches ; 6 x 12 = 72
8.) 36 inches = approximately 1 yard ; 36 x 0.027777 = .99....
9.) 12 yards = approximately 4 feet ; 12 x 0.33333 = 3.99....
10.) 80 inches = approximately 67 feet ; 80 x 0.83333 = 66.66....

The following links will help you practice with conversions:

1.) Practice worksheet with inches, feet, and yards: easy level
2.) Answer key to worksheet 1
3.) Worksheet 2 with inches, feet, and yards: medium level
4.) Answer key to worksheet 2

Measurement in Terms of Length: Metric System

Hello,

In our last post we learned what Fahrenheit and Celsius were.  Today, we are going to learn about the metric system and some of its prefixes.  The metric system is a system of measurement that involves decimals.  The original metric system was discovered in France around the 1790s. The metric system is usually abbreviated by SI.  In this post, we will go over some basic units of length, and how to convert units of measurement in terms of meters.  

The standard unit of length is the meter, abbreviated by m.  Other units of length include:

1.) Millimeter---> abbreviated by mm.
2.) Centimeter--->abbreviated by cm.
3.) Decimeter--->abbreviated by dm.
4.) Kilometer--->abbreviated by km.

Note:

1 millimeter = 0.001 meters; milli means thousandth
1 centimeter = 0.01 meters; centi means hundredth
1 kilometer = 1000 meters; kilo means thousand

Now, let's try converting measurements back into meters.

1.) 54.7 cm = ? m
2.) 23.8 mm = ? m
3.) 123 km = ? m

Solutions:

1.) Since 1 cm = 0.01 m, we must multiply (54.7 x 0.01) to find how many meters are in 54.7 centimeters.  54.7 x 0.01 = 0.547.  So, 54.7 cm = 0.547 m. 


2.) Since 1 mm = 0.001 m, multiply (23.8 x 0.001) to find how many meters are in 23.8 millimeters.  23.8 x 0.001 = 0.0238.  So, 23.8 mm = 0.0238 m.


3.) Since 1 km = 1000 m, multiply (123 x 1000) to find how many meters are in 123 kilometers.  123 x 1000 = 123,000.  So, 123 km = 123,000 m.  


The following are links that will help reiterate these metric units and will help you practice:


1.) This link reiterates the basic units of length
2.) Explanation of measuring length in the metric system: comes with a practice
3.) Practice worksheet with conversions
4.) Answer key to practice worksheet

I hope these links help!

Measurement: Fahrenheit and Celsius

Hello,


Today, we are going to move on from measuring time, and we are going to look at Fahrenheit and Celsius.  Fahrenheit and Celsius are temperature scales.  Gabriel Daniel was the inventor of Fahrenheit, and he discovered the mercury thermometer.  Did you know that water freezes at 32 degrees Fahrenheit and it boils at  212 degrees Fahrenheit?  We label Fahrenheit with an F and a degree symbol.  Celsius represents the temperature scale where 0 degrees is the freezing point and 100 degrees is the boiling point.  Anders Celsius invented the Celsius scale.  


We are going to practice converting Fahrenheit to Celsius and Celsius to Fahrenheit.  


In order to convert from Fahrenheit to Celsius, we must first note that there is a 180 degree difference between the Fahrenheit scale and Celsius scale.  Every degree on the Fahrenheit scale is 100/180, and if we reduce this, dividing each by 20, we get 5/9 on the Celsius scale.  To convert from Fahrenheit to Celsius, we first subtract 32 degrees from our temperature.  Second, we must multiply our answer by our 5/9 that we came up with before.     


In order to convert a temperature from Celsius to Fahrenheit, we must first note that every degree on the Celsius scale is opposite the Fahrenheit scale: 180/100 or 9/5.  So, basically, we are performing the opposite operation depending on which temperature scale we start with.  To convert from Celsius to Fahrenheit, we first multiply the Celsius temperature by our 9/5 from earlier.  Second, you must add 32 degrees.  


Let's try some examples of converting from Fahrenheit to Celsius:


1.) 67.3 degrees F = how many degrees C?
2.) 45.0 degrees F = how many degrees C?
3.) 89.1 degrees F = how many degrees C?


Solutions:


1.) Subtract 67.3-32.0 = 35.3.  Now, multiply this by 5/9.  35.3 x 5 = 176.5 / 9 = 19.61 degrees C, rounded to the nearest hundredth.  


2.) Subtract 45.0-32.0 = 13.  Now, multiply this by 5/9.  13 x 5 = 65 / 9 = 7.22 degrees C, to the nearest hundredth.


3.) Subtract 89.1-32.0 = 57.1.  Now, multiply this by 5/9. 57.1 x 5 = 285.5 / 9 = 31.72 degrees C, to the nearest hundredth.


Let's try some examples of converting from C to F now:


1.) 80.7 degrees C = how many degrees F?
2.) 12.7 degrees C = how many degrees F?
3.) 56.4 degrees C = how many degrees F?


Solutions: 


1.) Multiply 80.7 x 9/5 = 145.26.  Now, add 32 degrees.  145.26 + 32 = 177.26 degrees F.


2.) Multiply 12.7 x 9/5 = 22.86.  Now, add 32 degrees. 22.86 + 32 = 54.86 degrees F.


3.) Multiply 56.4 x 9/5 = 101.52.  Now, add 32 degrees.  101.52 + 32 = 133.52 degrees F.


The following links are useful for further explanation and self-converter of the temperature scales and conversions:


1.) Temperature Scale Converter: Should only be used to check your answers
2.) 3 Different Temperature Scales Used Today